Projects
About Us
More than 10 years of experience in engineering, applying synergy of several technical areas together with new technologies to guarantee the success of our customers.
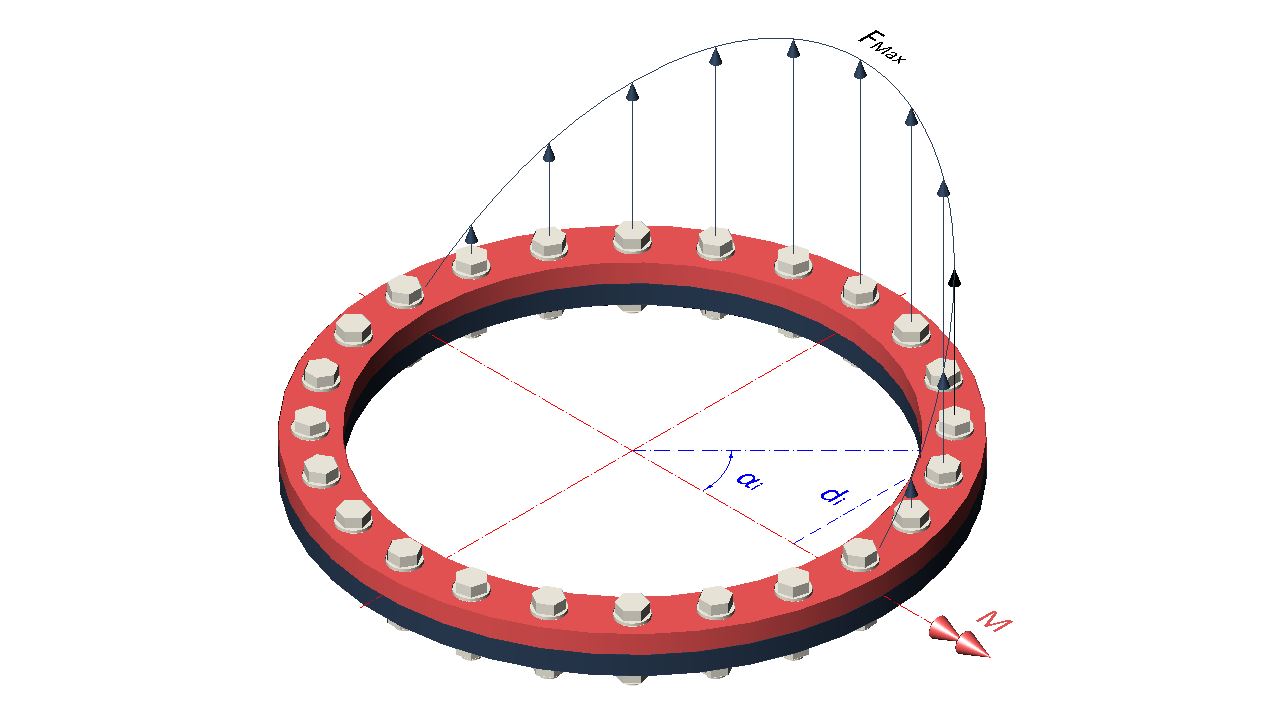
Bolted Circular Flange
In engineering, it is very common to find a circular flange fastened with bolts that is subjected to a load moment. This is the case of pipe joints or plates which support a bearing for rotary motion.
If there is a moment centered with the flange, given that the bolts of the joint are arranged at different distances from the moment axis, each of them will support a different tensile force, so it is necessary to determine these loads to size the bolted joint.
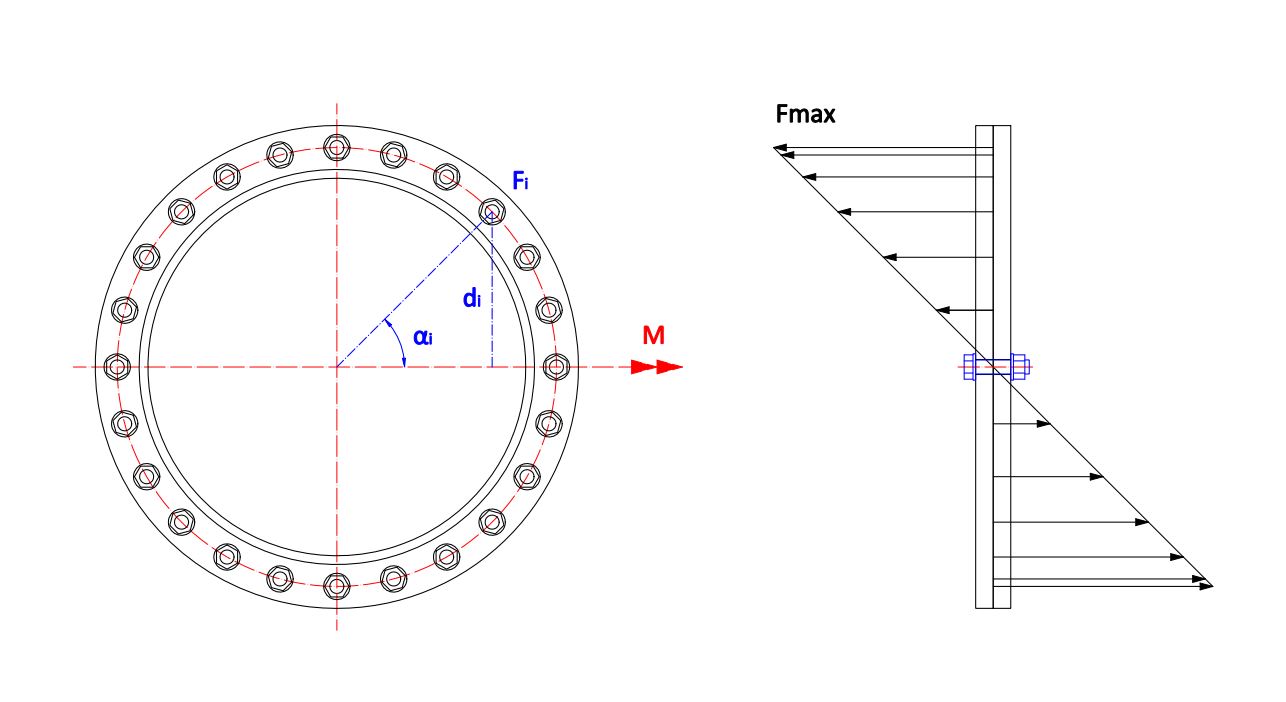
Load distribution
To begin with, the bolts farthest from the moment axis will support the maximum load (Fmax), which we will simply call F, while the bolts crossing the moment axis will have a zero tensile load.
The other bolt loads between these two positions depends on their distance from the moment axis in a linear fashion, if we look at the flange in profile, but their contribution to the moment depends circularly on the position they occupy on the flange circumference.
Load distribution scheme
Thus, the distance (di) from each bolt to the moment axis can be calculated as:
\normalsize d_i = R sin (\alpha_i)
Being R the bolted circumference radius and alpha the angle with regards to the moment axis.
And loads of each bolt can be calculated by similar triangles in the flange profile as:
\Large \frac {F}{R} = \frac {F_i}{d_i}
Where Fi is the load on each bolt, with the other variables already defined. By subtracting Fi we have that:
\normalsize F_i = F sin (\alpha_i)
The total moment on the flange (M) will be the sum of the force of each bolt multiplied by its distance to the moment axis, from i=0 to n
\normalsize M = \sum_{i=0}^n F sin (\alpha_i) \cdot R sin (\alpha_i) = F R \sum_{i=0}^n sin^2 (\alpha_i) = \Large \frac {F R n} {2}
Therefore, the maximum force on the bolt farthest from the moment axis will be:
F = \Large \frac {2 M} {n R}
And the forces on each screw will be the maximum force times the sine of the angle (alpha) which occupies on the circumference.
\normalsize F_i = F sin (\alpha_i) = \Large \frac {2 M} {n R} \normalsize sin (\alpha_i)
It should be noted that the moment on the flange affects the tensile bolts only half of it, since the other half, subjected to compression, tries to join both plates of the flange, so that the bolts in this area do not support loads due to the moment.
Mathematical Development
\normalsize M = \sum_{i=0}^n F sin (\alpha_i) \cdot R sin (\alpha_i) = FR \sum_{i=0}^n sin^2 (\alpha_i) =
(1) = FR \int_0^{2\pi} sin^2 (\alpha) \large \frac {n}{2\pi} \normalsize d\alpha = (2) = \frac {FRn}{2\pi} \int_0^{2\pi} \frac{1}{2}(1-cos (2\alpha)) \normalsize d\alpha =
= \frac {1}{2} \frac {FRn}{2\pi}\{ [\alpha ]_{0}^{\rm 2\pi} - \cancel{[\frac{1}{2} sin(2\alpha)]_{0}^{\rm 2\pi}}\} =\large \frac {FRn}{2}
\normalsize For i = 0 \longrightarrow \alpha = 0
\normalsize For i = n \longrightarrow \alpha = 2\pi
\alpha = \large \frac{2\pi}{n} \normalsize i
i = \large \frac{n}{2\pi} \normalsize \alpha ; di = \large \frac{n}{2\pi} \normalsize d\alpha
\normalsize sin^2 (\alpha) + cos^2 (\alpha) = 1
\normalsize sin^2 (\alpha) = 1 - cos^2 (\alpha) (A)
\normalsize cos (2\alpha) = cos^2 (\alpha) - sin^2 (\alpha)
\normalsize cos^2 (\alpha) = cos (2\alpha) - sin^2 (\alpha) (B)
Substituting B in A:
\normalsize sin^2 (\alpha) = \frac{1}{2} (1 - cos (2\alpha)) (2)
